- Professional Development
- Medicine & Nursing
- Arts & Crafts
- Health & Wellbeing
- Personal Development
Description Math is one of the most essential skills in all aspects of our lives. However, it can be one of the most difficult for many of us to master. Learning math can be a daunting task when you do not have the right tools or the right materials, especially if you grew up believing that math is hard and that you will never understand it. The Mathematics for Beginners is designed to teach the elementary skills necessary to manipulate algebraic expressions and equations, and also places a strong emphasis on conceptual understanding. You will learn the connection between everyday life problems and the abstract world of mathematics. Using applied examples from a wide variety of topics ranging from finance to physics and life sciences, you will gain the ability to transform problems from a verbal description into the language of mathematics and solve them confidently by applying the rules of algebra. Assessment: At the end of the course, you will be required to sit for an online MCQ test. Your test will be assessed automatically and immediately. You will instantly know whether you have been successful or not. Before sitting for your final exam you will have the opportunity to test your proficiency with a mock exam. Certification: After completing and passing the course successfully, you will be able to obtain an Accredited Certificate of Achievement. Certificates can be obtained either in hard copy at a cost of £39 or in PDF format at a cost of £24. Who is this Course for? Mathematics for Beginners is certified by CPD Qualifications Standards and CiQ. This makes it perfect for anyone trying to learn potential professional skills. As there is no experience and qualification required for this course, it is available for all students from any academic background. Requirements Our Mathematics for Beginners is fully compatible with any kind of device. Whether you are using Windows computer, Mac, smartphones or tablets, you will get the same experience while learning. Besides that, you will be able to access the course with any kind of internet connection from anywhere at any time without any kind of limitation. Career Path After completing this course you will be able to build up accurate knowledge and skills with proper confidence to enrich yourself and brighten up your career in the relevant job market. Integers ( Directed Numbers) Lecture 1 Introduction 00:02:00 Lecture 2 Quick glance at number system 00:05:00 Lecture 3 Integers and ordering of integers 00:06:00 Lecture 4 Addition and Subtractions of Integers 00:07:00 Lecture 5 Operations on Integers 00:04:00 Lecture 6 Multiplication and Division of Integers 00:04:00 Lecture 7 Powers of Integers (Exponents) 00:04:00 Factors and Multiples Lecture 8 Complete knowledge about factors and multiples 00:06:00 Lecture 9 Divisibility tests for 2,3,4,5,6 00:07:00 Lecture 10 Divisibility rules 7,8,9,10,11 00:06:00 Lecture 11 Prime Factorization 00:06:00 Lecture 12 Highest Common Factor HCF 00:10:00 Lecture 13 Least Common Multiple LCM 00:16:00 Lecture 14 Relation between LCM and HCF 00:04:00 Fractions Lecture 15 Classification of Fractions 00:04:00 Lecture 16 Convert mixed to improper and improper to mixed fractions 00:03:00 Lecture 17 Equivalent Fractions 00:04:00 Lecture 18 Comparing Fractions after converting to like fractions 00:09:00 Lecture 19 Reducing a fraction to lowest term 00:04:00 Lecture 20 Addition and subtraction OF fractions 00:08:00 Lecture 21 Multiplication and division of fractions 00:11:00 Lecture 22 Word problems on fractions 00:16:00 Simplification Rule : BODMAS Lecture 23 BODMAS and rules to remove brackets 00:03:00 Lecture 24 Simplify expressions using BODMAS rules 00:05:00 Lecture 25 Simplify expressions involving fractions using BODMAS 00:07:00 Decimal numbers Lecture 26 Decimal numbers in relation to fractions 00:08:00 Lecture 27 Like and unlike Decimals 00:05:00 Lecture 28 Ordering of Decimal numbers 00:10:00 Lecture 29 Addition and subtraction of Decimal 00:07:00 Lecture 30 Multiplication of Decimal 00:03:00 Lecture 31 Division of Decimal 00:05:00 Rational Numbers Lecture 32 Rational number 00:02:00 Lecture 33 Representation of Rational Numbers on Number line 00:04:00 Lecture 34 Determining which fraction is terminating or non terminating 00:05:00 Lecture 35 shortcut method of writing decimal expansion of fraction to decimal 00:08:00 Approximation Lecture 36 Rounding to whole number 00:06:00 Lecture 37 Rounding to required number of decimals (1 d.p. ) 00:05:00 Lecture 38 rounding to significant digits 00:07:00 Lecture 39 Practice question on three methods of approximation 00:05:00 Estimation and Scientific Notation or Standard form Lecture 40 Estimation 00:05:00 Lecture 41 Scientific Notation 00:07:00 Lecture 42 Operation on numbers when they are in scientific notation 00:06:00 Percentage Lecture 43 Percentage to fraction and fraction to percentage 00:06:00 Lecture 44 Percentage of a quantity and conversation to decimal 00:06:00 Lecture 45 Expressing one quantity as percentage of another quantity 00:06:00 Lecture 46 Finding increase decrease percent 00:05:00 Lecture 47 Uses of percentages-Word Problems 00:09:00 Ratio and Proportion Lecture 48 Ratio- How to express as simple ratio 00:09:00 Lecture 49 How to compare Ratios 00:04:00 Lecture 50 Word problems on finding ratios 00:07:00 Lecture 51To divide a given quantity into a given Ratio 00:11:00 Lecture 52 Proportion 00:04:00 Lecture 53 Practice problems on Proportion 00:06:00 Lecture 54 Continued proportion 00:06:00 Unitary Method and its Applications Lecture 55 Direct Variation ( or proportion) 00:04:00 Lecture 56 Problems based on Direct proportion 00:10:00 Lecture 57 Inverse Variation ( or proportion) 00:10:00 Lecture 58 Multiple Ratios 00:10:00 Profit , Loss, discount and Tax Lecture 59 Basics - Profit and Loss 00:04:00 Lecture 60 More practice problems on Profit & Loss 00:06:00 Lecture 61 Selling price formula and Problems 00:04:00 Lecture 62 Cost price formula and Problems 00:03:00 Lecture 63 Higher problems on Profit and Loss 00:08:00 Lecture 64 Basics - Discount.mp4 00:05:00 Lecture 65 Practice problems on Discount 00:04:00 Lecture 66 Tax 00:06:00 Resources Resources - Mathematics for Beginners 00:00:00 Mock Exam Mock Exam - Mathematics for Beginners 00:20:00 Final Exam Final Exam - Mathematics for Beginners 00:20:00 Order Your Certificates and Transcripts Order Your Certificates and Transcripts 00:00:00

QUALIFI Level 3 Diploma in Data Science
By School of Business and Technology London
Getting Started The QUALIFI Level 3 Diploma in Data Science aims to offer learners a comprehensive introduction to data science. This Level 3 Diploma provides a modern and all-encompassing overview of data science, artificial intelligence, and machine learning. It covers the evolution of artificial intelligence and machine learning from their beginnings in the late 1950s to the emergence of the "big data" era in the early 2000s. It extends to the current AI and machine learning applications, including the associated challenges. In addition to covering standard machine learning models like linear and logistic regression, decision trees, and k-means clustering, this diploma introduces learners to two emerging areas of data science: synthetic data and graph data science. Moreover, the diploma familiarizes learners with the landscape of data analysis and the relevant analytical tools. It includes introducing Python programming so learners can effectively analyse, explore, and visualize data and implement fundamental data science models. Key Benefits Acquire the essential mathematical and statistical knowledge necessary for conducting fundamental data analysis. Cultivate analytical and machine learning proficiency using Python. Foster a solid grasp of data and its related processes, encompassing data cleaning, data structuring, and data preparation for analysis and visualisation. Gain insight into the expansive data science landscape and ecosystem, including relational databases, graph databases, programming languages like Python, visualisation tools, and various analytical instruments. Develop expertise in comprehending the machine learning procedures, including the ability to discern which algorithms are suited for distinct problems and to navigate the steps involved in constructing, testing, and validating a model. Attain an understanding of contemporary and emerging facets of data science and their applicability to modern challenges Key Highlights This course module prepares learners for higher-level Data science positions through personal and professional development. We will ensure your access to the first-class education needed to achieve your goals and dreams and to maximize future opportunities. Remember! The assessment for the Qualification is done based on assignments only, and you do not need to worry about writing any exam. With the School of Business and Technology London, you can complete the Qualification at your own pace, choosing online or blended learning from the comfort of your home. Learning and pathway materials and study guides developed by our qualified tutors will be available around the clock in our cutting-edge learning management system. Most importantly, at the School of Business and Technology London, we will provide comprehensive tutor support through our dedicated support desk. If you choose your course with blended learning, you will also enjoy live sessions with an assigned tutor, which you can book at your convenience. Career Pathways Upon completing the QUALIFI Level 3 Diploma in Data Science, learners can advance their studies or pursue employment opportunities. Data Analyst with an estimated average salary of £39,445 per annum Business Intelligence Analyst with an estimated average salary of £40,000 per annum Data entry specialist with an estimated average salary of £22,425 per annum Database Administrator with an estimated average salary of £44,185 per annum About Awarding Body QUALIFI, recognised by Ofqual awarding organisation has assembled a reputation for maintaining significant skills in a wide range of job roles and industries which comprises Leadership, Hospitality & Catering, Health and Social Care, Enterprise and Management, Process Outsourcing and Public Services. They are liable for awarding organisations and thereby ensuring quality assurance in Wales and Northern Ireland. What is included? Outstanding tutor support that gives you supportive guidance all through the course accomplishment through the SBTL Support Desk Portal. Access our cutting-edge learning management platform to access vital learning resources and communicate with the support desk team. Quality learning materials such as structured lecture notes, study guides, and practical applications, which include real-world examples and case studies, will enable you to apply your knowledge. Learning materials are provided in one of the three formats: PDF, PowerPoint, or Interactive Text Content on the learning portal. The tutors will provide Formative assessment feedback to improve the learners' achievements. Assessment materials are accessible through our online learning platform. Supervision for all modules. Multiplatform accessibility through an online learning platform facilitates SBTL in providing learners with course materials directly through smartphones, laptops, tablets or desktops, allowing students to study at their convenience. Live Classes (for Blended Learning Students only) Assessment Time-constrained scenario-based assignments No examinations Entry Requirements The qualification has been intentionally designed to ensure accessibility without imposing artificial barriers that limit entry. To enrol in this qualification, applicants must be 18 years of age or older. Admittance to the qualification will be managed through centre-led registration processes, which may involve interviews or other appropriate procedures. Despite the presence of advanced mathematics and statistics in higher-level data science courses, encompassing subjects such as linear algebra and differential calculus, this Level 3 Diploma only requires learners to be comfortable with mathematics at the GCSE level. The diploma's mathematical and statistical concepts are based on standard mathematical operations like addition, multiplication, and division. Before commencing the Level 3 Diploma in Data Science, learners are expected to meet the following minimum requirements: i) GCSE Mathematics with a grade of B or higher (equivalent to the new level 6 or above); and ii) GCSE English with a grade of C or higher (equivalent to the new level 4 or above). Furthermore, prior coding experience is not mandatory, although learners should be willing and comfortable with learning Python. Python has been selected for its user-friendly and easily learnable nature. In exceptional circumstances, applicants with substantial experience but lacking formal qualifications may be considered for admission, contingent upon completing an interview and demonstrating their ability to meet the demands of the capability. Progression Upon successful completion of the QUALIFI Level 3 Diploma in Data Science, learners will have several opportunities: Progress to QUALIFI Level 4 Diploma in Data Science: Graduates can advance their education and skills by enrolling in the QUALIFI Level 4 Diploma in Data Science, which offers a more advanced and comprehensive study of the field. Apply for Entry to a UK University for an Undergraduate Degree: This qualification opens doors to higher education, allowing learners to apply for entry to a UK university to pursue an undergraduate degree in a related field, such as data science, computer science, or a related discipline. Progress to Employment in an Associated Profession: Graduates of this program can enter the workforce and seek employment opportunities in professions related to data science, artificial intelligence, machine learning, data analysis, and other relevant fields. These progression options provide learners with a diverse range of opportunities for further education, career advancement, and professional development in the dynamic and rapidly evolving field of data science Why gain a QUALIFI Qualification? This suite of qualifications provides enormous opportunities to learners seeking career and professional development. The highlighting factor of this qualification is that: The learners attain career path support who wish to pursue their career in their denominated sectors; It helps provide a deep understanding of the health and social care sector and managing the organisations, which will, in turn, help enhance the learner's insight into their chosen sector. The qualification provides a real combination of disciplines and skills development opportunities. The Learners attain in-depth awareness concerning the organisation's functioning, aims and processes. They can also explore ways to respond positively to this challenging and complex health and social care environment. The learners will be introduced to managing the wide range of health and social care functions using theory, practice sessions and models that provide valuable knowledge. As a part of this suite of qualifications, the learners will be able to explore and attain hands-on training and experience in this field. Learners also acquire the ability to face and solve issues then and there by exposure to all the Units. The qualification will also help to Apply scientific and evaluative methods to develop those skills. Find out threats and opportunities. Develop knowledge in managerial, organisational and environmental issues. Develop and empower critical thinking and innovativeness to handle problems and difficulties. Practice judgement, own and take responsibility for decisions and actions. Develop the capacity to perceive and reflect on individual learning and improve their social and other transferable aptitudes and skills Learners must request before enrolment to interchange unit(s) other than the preselected units shown in the SBTL website because we need to make sure the availability of learning materials for the requested unit(s). SBTL will reject an application if the learning materials for the requested interchange unit(s) are unavailable. Learners are not allowed to make any request to interchange unit(s) once enrolment is complete. UNIT1- The Field of Data Science Reference No : H/650/4951 Credit : 6 || TQT : 60 This unit provides learners with an introduction to the field of data science, tracing its origins from the emergence of artificial intelligence and machine learning in the late 1950s, through the advent of the "big data" era in the early 2000s, to its contemporary applications in AI, machine learning, and deep learning, along with the associated challenges. UNIT2- Python for Data Science Reference No : J/650/4952 Credit : 9 || TQT : 90 This unit offers learners an introductory approach to Python programming tailored for data science. It begins by assuming no prior coding knowledge or familiarity with Python and proceeds to elucidate Python's fundamentals, including its design philosophy, syntax, naming conventions, and coding standards. UNIT3- Creating and Interpreting Visualisations in Data Science Reference No : K/650/4953 Credit : 3 || TQT : 30 This unit initiates learners into the realm of fundamental charts and visualisations, teaching them the art of creating and comprehending these graphical representations. It commences by elucidating the significance of visualisations in data comprehension and discerns the characteristics distinguishing effective visualisations from subpar ones. UNIT4- Data and Descriptive Statistics in Data Science Reference No : L/650/4954 Credit : 6 || TQT : 60 The primary objective of this unit is to acquaint learners with the foundational concepts of descriptive statistics and essential methods crucial for data analysis and data science. UNIT5- Fundamentals of Data Analytics Reference No : M/650/4955 Credit : 3 || TQT : 30 This unit will enable learners to distinguish between the roles of a Data Analyst, Data Scientist, and Data Engineer. Additionally, learners can provide an overview of the data ecosystem, encompassing databases and data warehouses, and gain familiarity with prominent vendors and diverse tools within this data ecosystem. UNIT6- Data Analysis with Python Reference No : R/650/4956 Credit : 3 || TQT : 30 This unit initiates learners into the fundamentals of data analysis using Python. It acquaints them with essential concepts like Pandas Data Frames and Series and the techniques of merging and joining data. UNIT7- Data Analysis with Python Reference No : R/650/4956 Credit : 3 || TQT : 30 This unit initiates learners into the fundamentals of data analysis using Python. It acquaints them with essential concepts like Pandas Data Frames and Series and the techniques of merging and joining data. UNIT8- Machine Learning Methods and Models in Data Science Reference No : T/650/4957 Credit : 3 || TQT : 30 This unit explores the practical applications of various methods in addressing real-world problems. It provides a summary of the key features of these different methods and highlights the challenges associated with each of them. UNIT9- The Machine Learning Process Reference No : Y/650/4958 Credit : 3 || TQT : 30 This unit provides an introduction to the numerous steps and procedures integral to the construction and assessment of machine learning models. UNIT10- Linear Regression in Data Science Reference No : A/650/4959 Credit : 3 || TQT : 30 This unit offers a foundational understanding of simple linear regression models, a crucial concept for predicting the value of one continuous variable based on another. Learners will gain the capability to estimate the best-fit line by computing regression parameters and comprehend the accuracy associated with this line of best-fit. UNIT11- Logistic Regression in Data Science Reference No : H/650/4960 Credit : 3 || TQT : 30 This unit introduces logistic regression, emphasizing its role as a classification algorithm. It delves into the fundamentals of binary logistic regression, covering essential concepts such as the logistic function, Odds ratio, and the Logit function. UNIT12- Decision Trees in Data Science Reference No : J/650/4961 Credit : 3 || TQT : 30 This unit offers an introductory exploration of decision trees' fundamental theory and practical application. It elucidates the process of constructing basic classification trees employing the standard ID3 decision-tree construction algorithm, including the node-splitting criteria based on information theory principles such as Entropy and Information Gain. Additionally, learners will gain hands-on experience in building and assessing decision tree models using Python. UNIT13- K-means clustering in Data Science Reference No : K/650/4962 Credit : 3 || TQT : 30 This unit initiates learners into unsupervised machine learning, focusing on the k-means clustering algorithm. It aims to give learners an intuitive understanding of the k-means clustering method and equip them with the skills to determine the optimal number of clusters. UNIT14- Synthetic Data for Privacy and Security in Data Science Reference No : L/650/4963 Credit : 6 || TQT : 60 This unit is designed to introduce learners to the emerging field of data science, specifically focusing on synthetic data and its applications in enhancing data privacy and security. UNIT15- Graphs and Graph Data Science Reference No : M/650/4964 Credit : 6 || TQT : 60 This unit offers a beginner-friendly introduction to graph theory, a foundational concept that underlies modern graph databases and graph analytics. Delivery Methods School of Business & Technology London provides various flexible delivery methods to its learners, including online learning and blended learning. Thus, learners can choose the mode of study as per their choice and convenience. The program is self-paced and accomplished through our cutting-edge Learning Management System. Learners can interact with tutors by messaging through the SBTL Support Desk Portal System to discuss the course materials, get guidance and assistance and request assessment feedbacks on assignments. We at SBTL offer outstanding support and infrastructure for both online and blended learning. We indeed pursue an innovative learning approach where traditional regular classroom-based learning is replaced by web-based learning and incredibly high support level. Learners enrolled at SBTL are allocated a dedicated tutor, whether online or blended learning, who provide learners with comprehensive guidance and support from start to finish. The significant difference between blended learning and online learning methods at SBTL is the Block Delivery of Online Live Sessions. Learners enrolled at SBTL on blended learning are offered a block delivery of online live sessions, which can be booked in advance on their convenience at additional cost. These live sessions are relevant to the learners' program of study and aim to enhance the student's comprehension of research, methodology and other essential study skills. We try to make these live sessions as communicating as possible by providing interactive activities and presentations. Resources and Support School of Business & Technology London is dedicated to offering excellent support on every step of your learning journey. School of Business & Technology London occupies a centralised tutor support desk portal. Our support team liaises with both tutors and learners to provide guidance, assessment feedback, and any other study support adequately and promptly. Once a learner raises a support request through the support desk portal (Be it for guidance, assessment feedback or any additional assistance), one of the support team members assign the relevant to request to an allocated tutor. As soon as the support receives a response from the allocated tutor, it will be made available to the learner in the portal. The support desk system is in place to assist the learners adequately and streamline all the support processes efficiently. Quality learning materials made by industry experts is a significant competitive edge of the School of Business & Technology London. Quality learning materials comprised of structured lecture notes, study guides, practical applications which includes real-world examples, and case studies that will enable you to apply your knowledge. Learning materials are provided in one of the three formats, such as PDF, PowerPoint, or Interactive Text Content on the learning portal. How does the Online Learning work at SBTL? We at SBTL follow a unique approach which differentiates us from other institutions. Indeed, we have taken distance education to a new phase where the support level is incredibly high.Now a days, convenience, flexibility and user-friendliness outweigh demands. Today, the transition from traditional classroom-based learning to online platforms is a significant result of these specifications. In this context, a crucial role played by online learning by leveraging the opportunities for convenience and easier access. It benefits the people who want to enhance their career, life and education in parallel streams. SBTL's simplified online learning facilitates an individual to progress towards the accomplishment of higher career growth without stress and dilemmas. How will you study online? With the School of Business & Technology London, you can study wherever you are. You finish your program with the utmost flexibility. You will be provided with comprehensive tutor support online through SBTL Support Desk portal. How will I get tutor support online? School of Business & Technology London occupies a centralised tutor support desk portal, through which our support team liaise with both tutors and learners to provide guidance, assessment feedback, and any other study support adequately and promptly. Once a learner raises a support request through the support desk portal (Be it for guidance, assessment feedback or any additional assistance), one of the support team members assign the relevant to request to an allocated tutor. As soon as the support receive a response from the allocated tutor, it will be made available to the learner in the portal. The support desk system is in place to assist the learners adequately and to streamline all the support process efficiently. Learners should expect to receive a response on queries like guidance and assistance within 1 - 2 working days. However, if the support request is for assessment feedback, learners will receive the reply with feedback as per the time frame outlined in the Assessment Feedback Policy.

Functional Skills Mini Bundle
By Compete High
The Functional Skills Mini Bundle delivers straightforward knowledge across essential topics—maths, English, IT, Microsoft Project, and self-organisation. If you've ever wondered when you’d use algebra again or struggled with that oddly formatted email, this one’s for you (and your future spreadsheet). It’s smart, clear and focused—perfect for building a solid academic and administrative foundation. Whether you’re brushing up on core subjects or preparing to be more organised than your calendar suggests, this bundle covers essential functions that play well in almost every environment—digital or otherwise. Learning Outcomes: Improve English reading, writing, and structured communication skills. Gain confidence in everyday numeracy and problem-solving concepts. Learn IT fundamentals with a focus on usability and clarity. Explore Microsoft Project structure and time-based task allocation. Understand how to prioritise tasks and meet planned objectives. Strengthen essential workplace and study-related digital skills. Who is this Course For: Job seekers needing a refresher on key academic subjects. Students aiming to build confidence in English and maths. Individuals looking to manage time and tasks more effectively. Beginners exploring the basics of information technology. Professionals wanting clarity in project management software. Anyone needing structured learning across core skill areas. Career changers preparing for office-based digital roles. Learners interested in self-organisation and efficient planning. Career Path: Administrative Assistant – £24,000/year Project Coordinator – £29,000/year Data Entry Officer – £23,500/year IT Support Assistant – £26,000/year Office Manager – £31,500/year Customer Service Executive – £25,000/year

Description Do you want to know the fundamental concepts of bonds including notes payable, bond payable, and liabilities along with its record procedure? Are you looking for any course regarding this topic? If so, look at our Accounting Bonds and Liabilities Training course. It will assist you in understanding all you need to know about this field. The course introduces you to different types of bond and their features and how to issue bonds according to par value, premium, and discount for the journal entry. It teaches you how to calculate different confusing present value in various effective ways using value table, Microsoft Excel functions, and algebra and formula. The course will enable you to identify the reasons of fluctuation of price value, know amortization tables for accurate transactions on a note payable bond. It also teaches you how to create a liability section of the balance sheet, differentiates between capital lease and operating lease, and solve the problems stepping back and thinking about the whole accounting phases. Assessment: This course does not involve any MCQ test. Students need to answer assignment questions to complete the course, the answers will be in the form of written work in pdf or word. Students can write the answers in their own time. Once the answers are submitted, the instructor will check and assess the work. Certification: After completing and passing the course successfully, you will be able to obtain an Accredited Certificate of Achievement. Certificates can be obtained either in hard copy at a cost of £39 or in PDF format at a cost of £24. Who is this Course for? Accounting Bonds and Liabilities Training is certified by CPD Qualifications Standards and CiQ. This makes it perfect for anyone trying to learn potential professional skills. As there is no experience and qualification required for this course, it is available for all students from any academic background. Requirements Our Accounting Bonds and Liabilities Training is fully compatible with any kind of device. Whether you are using Windows computer, Mac, smartphones or tablets, you will get the same experience while learning. Besides that, you will be able to access the course with any kind of internet connection from anywhere at any time without any kind of limitation. Career Path After completing this course you will be able to build up accurate knowledge and skills with proper confidence to enrich yourself and brighten up your career in the relevant job market. Introduction Introduction 00:08:00 PDF - 10 Bond & Note Payable Introduction 00:01:00 10 Bonds & Notes Payable Introduction 00:10:00 Accounting Comic Break 00:01:00 Bonds - Market Rate vs Contract Rate 2 Bonds Market Rate vs Contract Rate 00:01:00 PDF - 20 Bonds Issued at Par 00:01:00 20 Bond Issued at Par 00:06:00 PDF - 25 Bond Market Price vs Contract Rate 00:01:00 Excel Download 00:01:00 Worksheet - 20 Bond Issued at Par 00:06:00 Excel Download 00:01:00 Bonds Issued at Premium 3 Bonds Issued at Premium 00:01:00 PDF - 50 Bond Issued at Premium 00:08:00 50 Bond Issued at Premium 00:07:00 Excel Download 00:01:00 Worksheet - 50 Bond Issue at Premium 00:09:00 PDF - 60 Amortization Premium & Recording Interest 00:01:00 60 Premium Amortization & Interest 00:13:00 Excel Download 00:01:00 Worksheet - 55 Bond Premium and Interest Journal Entry 00:16:00 10 Multiple Choice Question - Long Term Liabilities 00:07:00 Accounting Comic Break 00:01:00 Bonds Issued at Discount 4 Bonds Issued at Discount 00:01:00 PDF - 30 Bonds Issued at Discount 00:01:00 PDF - 40 Amortizing Discount & Recording Interest 00:01:00 40 Issue bond at a discount%2C calculate%2C and record interest 00:26:00 Excel Download 00:01:00 Worksheet - 30 Bond Issued at Discount 00:09:00 Excel Download 00:01:00 Worksheet - 35 Bond Discount & Interest 00:18:00 Excel Download 00:01:00 Worksheet - 1400.10 Record issuance of bond at a discount amortiz 00:16:00 Worksheet - 1400.20 Record issuance of bond at a discount amortiz 00:16:00 Worksheet - 1400.40 Record issuance of bond at a premium amortize 00:16:00 Worksheet - 1400.50 Record issuance of bond at a premium amort. 00:14:00 20 Multiple Choice Question - Long Term Liabilities 00:04:00 Accounting Comic Break 00:01:00 Present Value - Bond Price 5 Present Value Bond Price 00:03:00 PDF - 70 Bond Present Value Formula 00:14:00 Excel Download 00:01:00 Worksheet - 70 Bond Present Value Formula 00:15:00 PDF - 80 Bond Present Value Tables 00:01:00 80 Bond Present Value Tablesy 00:14:00 Excel Download 00:01:00 Worksheet - 80 Bond Present Value Tables 00:11:00 Excel Download 00:01:00 Worksheet - 90 Bond Present Value Excel 00:20:00 30 Multiple Choice Question - Long Term Liabilities 00:07:00 Accounting Comic Break 00:01:00 Bond Retirement 6 Bond Retirement 00:01:00 PDF - 100 Bond Retirement 00:01:00 100 Bond Retirement 00:09:00 Excel Download 00:01:00 Worksheet - 100 Bond Retirement 00:12:00 40 Multiple Choice Question - Long Term Liabilities 00:07:00 Accounting Comic Break 00:01:00 Notes Payable Introduction 7 Notes Payable Introduction 00:01:00 PDF - 110 Notes Payable Introduction 00:01:00 110 Notes Payable Introduction 00:05:00 PDF - 120 Installment Note Journal Entry 00:01:00 Excel Download 00:01:00 50 Multiple Choice Question - Long Term Liabilities 00:05:00 Accounting Comic Break 00:01:00 Amortization Schedule - Notes Payable 8 Amortization Schedule Notes Payable 00:01:00 PDF - 130 Notes Payable Amortization 00:01:00 130 Amortization Schedule 00:12:00 PDF - 140 Notes Payable Interest Payments 00:01:00 140 Notes Payable Payments Journal Entry 00:07:00 Excel Download 00:01:00 Worksheet - 130 Note Payable Amortization 00:15:00 Excel Download 00:01:00 Worksheet - 140 Note payable interest payments 00:10:00 60 Multiple Choice Question - Long Term Liabilities 00:06:00 Accounting Comic Break 00:01:00 Notes Payable Adjusting Entries 9 Notes Payable Adjusting Entries 00:01:00 PDF - 150 Adjusting Entry - Notes Payable 00:01:00 150 Notes Payable Adjusting Entry 00:10:00 Excel Download 00:01:00 Worksheet - 150 Note Payable Adjusting Entry 1 00:07:00 Excel Download 00:01:00 Worksheet - 160 Note Payable Adjusting Entry 2 00:06:00 70 Multiple Choice Question - Long Term Liabilities 00:07:00 Accounting Comic Break 00:01:00 Financial Statements - Long Term Liabilities 10 Financial Statements Long Term Liabilities 00:01:00 PDF - 170 Liabilities - Current vs Non Current 00:01:00 170 Notes Payable Current vs. Non Current 00:18:00 Excel Download 00:01:00 Worksheet - 170 FS St LT One Loan 1 TB Account 00:09:00 Excel Download 00:01:00 Worksheet - 180 FS St LT Loan 1 loan 2 TB Accounts 00:08:00 Excel Download 00:01:00 Worksheet - 190 FS ST LT 2 loans 1 TB account 00:08:00 Excel Download 00:01:00 Worksheet - 200 FS ST LT 2 Loans 1 ST 1 LT TB account 00:09:00 Excel Download 00:01:00 Worksheet - 210 FS ST LT 2 Loans 2 Loan Account TB 00:08:00 Excel Download 00:01:00 Worksheet - 220 FS 2 Loans 4 Accounts TB 00:11:00 80 Multiple Choice Question - Long Term Liabilities 00:07:00 Accounting Comic Break 00:01:00 Bond Characteristics and Types 11 Bond Characteristics and Types 00:01:00 PDF - 230 Bond types 00:01:00 230 Bond Characteristics 00:02:00 90 Multiple Choice Question - Long Term Liabilities 00:05:00 Short Calculation 00:08:00 Accounting Comic Break 00:01:00 Effective Method - Amortization Bond Discount & Premium 12 Effective Method Amortization Bond Discount & Premium 00:01:00 PDF - 230 Effective Interest Discount Amortization 00:01:00 235 Discount Amortization Effective Method 00:13:00 Excel Download 00:01:00 Worksheet - 230 Effective Interest Discount Amortization 00:17:00 Excel Download 00:01:00 Worksheet - 240 Effective Interest Premium Amortization 00:13:00 100 Multiple Choice Question - Long Term Liabilities 00:10:00 Leases - Operating vs. Capital 13 Leases Operating vs. Capital 00:01:00 PDF - 250 Leases 00:01:00 250 Leases Capital vs. Operating 00:12:00 Accounting Comic Break 00:01:00 Comprehensive Problem 14 Comprehensive Problem 00:01:00 Excel Download 00:01:00 1 Accounting%2C Financial - Comp Prob Service Co 1 Part 1 00:15:00 2 Accounting%2C Financial - Comp Prob Service Co 1 Part 2 00:15:00 3 Accounting%2C Financial - Comp Prob Service Co 1 Part 3 00:15:00 4 Accounting%2C Financial - Comp Prob Service Co 1 Part 4_2 00:22:00 6 Comp Prob Service Co 1 Adjusting Entries part 6 00:20:00 Definitions & Key Terms 15 Definitions & Key Terms 00:01:00 Annuity Definition - What is Annuity 00:05:00 Bond Definition - What is Bond 00:06:00 Book Value of Bonds - What is Book Value of Bonds 00:06:00 Carrying Value of Bonds Definition - What is Carrying Value 00:05:00 Lease Definition - What is a Lease 00:01:00 Certificate and Transcript Order Your Certificates and Transcripts 00:00:00

Create a Compelling Competitive Matrix
By The Teachers Training
Learn to develop a compelling competitive matrix with our expert-led course. Gain insights into analyzing competitors, identifying strengths and weaknesses, and crafting effective strategies to gain a competitive edge. Enroll now to sharpen your business acumen and drive success in your industry.

Linear Regression Analysis in Microsoft Excel
By Study Plex
Recognised Accreditation This course is accredited by continuing professional development (CPD). CPD UK is globally recognised by employers, professional organisations, and academic institutions, thus a certificate from CPD Certification Service creates value towards your professional goal and achievement. The Quality Licence Scheme is a brand of the Skills and Education Group, a leading national awarding organisation for providing high-quality vocational qualifications across a wide range of industries. What is CPD? Employers, professional organisations, and academic institutions all recognise CPD, therefore a credential from CPD Certification Service adds value to your professional goals and achievements. Benefits of CPD Improve your employment prospects Boost your job satisfaction Promotes career advancement Enhances your CV Provides you with a competitive edge in the job market Demonstrate your dedication Showcases your professional capabilities What is IPHM? The IPHM is an Accreditation Board that provides Training Providers with international and global accreditation. The Practitioners of Holistic Medicine (IPHM) accreditation is a guarantee of quality and skill. Benefits of IPHM It will help you establish a positive reputation in your chosen field You can join a network and community of successful therapists that are dedicated to providing excellent care to their client You can flaunt this accreditation in your CV It is a worldwide recognised accreditation What is Quality Licence Scheme? This course is endorsed by the Quality Licence Scheme for its high-quality, non-regulated provision and training programmes. The Quality Licence Scheme is a brand of the Skills and Education Group, a leading national awarding organisation for providing high-quality vocational qualifications across a wide range of industries. Benefits of Quality License Scheme Certificate is valuable Provides a competitive edge in your career It will make your CV stand out Course Curriculum Getting Data Ready for Regression Model Transportation Problem in Excel using Goal Seek 00:12:00 Gathering Business Knowledge 00:03:00 Data Exploration 00:03:00 The Data and the Data Dictionary 00:07:00 Univariate analysis and EDD 00:03:00 Discriptive Data Analytics in Excel 00:10:00 Outlier Treatment 00:04:00 Identifying and Treating Outliers in Excel 00:04:00 Missing Value Imputation 00:03:00 Identifying and Treating missing values in Excel 00:04:00 Variable Transformation in Excel 00:03:00 Dummy variable creation: Handling qualitative data 00:04:00 Dummy Variable Creation in Excel 00:07:00 Correlation Analysis 00:09:00 Creating Correlation Matrix in Excel 00:08:00 Creating Regression Model The Problem Statement 00:01:00 Basic Equations and Ordinary Least Squares (OLS) method 00:08:00 Assessing accuracy of predicted coefficients 00:14:00 Assessing Model Accuracy: RSE and R squared 00:07:00 Creating Simple Linear Regression model 00:02:00 Multiple Linear Regression 00:05:00 The F - statistic 00:08:00 Interpreting results of Categorical variables 00:05:00 Creating Multiple Linear Regression model 00:07:00 What-if analysis Excel: Running Linear Regression using Solver 00:08:00 Assessment Assessment - Linear Regression Analysis In MS Excel 00:10:00 Certificate of Achievement Certificate of Achievement 00:00:00 Get Your Insurance Now Get Your Insurance Now 00:00:00 Feedback Feedback 00:00:00

Overview This comprehensive course on Pure Mathematics Fundamentals will deepen your understanding on this topic. After successful completion of this course you can acquire the required skills in this sector. This Pure Mathematics Fundamentals comes with accredited certification from CPD, which will enhance your CV and make you worthy in the job market. So enrol in this course today to fast track your career ladder. How will I get my certificate? You may have to take a quiz or a written test online during or after the course. After successfully completing the course, you will be eligible for the certificate. Who is This course for? There is no experience or previous qualifications required for enrolment on this Pure Mathematics Fundamentals. It is available to all students, of all academic backgrounds. Requirements Our Pure Mathematics Fundamentals is fully compatible with PC's, Mac's, Laptop, Tablet and Smartphone devices. This course has been designed to be fully compatible with tablets and smartphones so you can access your course on Wi-Fi, 3G or 4G. There is no time limit for completing this course, it can be studied in your own time at your own pace. Career Path Learning this new skill will help you to advance in your career. It will diversify your job options and help you develop new techniques to keep up with the fast-changing world. This skillset will help you to- Open doors of opportunities Increase your adaptability Keep you relevant Boost confidence And much more! Course Curriculum 14 sections • 193 lectures • 03:43:00 total length •About Course: 00:02:00 •Quick Guide: 00:01:00 •Topics of Essential Revision - 1: 00:00:00 •Negative numbers and operations on Integers: 00:14:00 •The rules of Indices in Algebra: 00:11:00 •Working with indices Part 1: 00:10:00 •Working with indices Part 2: 00:08:00 •Fractional Indices: 00:12:00 •What are Polynomials?: 00:07:00 •Writing statements in Algebraic Form: 00:06:00 •Simplification using BODMAS: 00:08:00 •Distributive Property: 00:07:00 •Addition of Algebraic expressions: 00:13:00 •Subtraction of Algebraic expressions: 00:12:00 •Multiplication of Algebraic Expressions Part 1: 00:05:00 •Multiplication of Algebraic Expressions Part 2: 00:05:00 •Multiplication of Algebraic Expressions Part 3: 00:06:00 •Division of algebraic expressions Part 1: 00:11:00 •Division of algebraic expressions Part 2: 00:10:00 •Division of algebraic expressions Part 3: 00:07:00 •Topics of Essential Revision - 2: 00:00:00 •Factorization by method of common factor: 00:13:00 •Factorization by regrouping the terms: 00:10:00 •Factorization by difference of two squares: 00:11:00 •Factorization using identity (a + b) ² and (a - b) ²: 00:10:00 •Factorization using identity (a + b + c) ²: 00:05:00 •Factorization by middle term split Part 1: 00:12:00 •Factorization by middle term split Part 2: 00:09:00 •Simultaneous Linear Equations: 00:07:00 •Graphical Method: 00:06:00 •Graphical method Continued: 00:11:00 •Elimination by substitution Method: 00:09:00 •Equating the coefficients Method: 00:11:00 •Cross Multiplication: 00:10:00 •Equations Reducible to Linear Equations-1: 00:08:00 •Equations Reducible to Linear Equations-2: 00:14:00 •Introduction to Quadratic Equations: 00:05:00 •Solving Quadratic Equations by Factorization method: 00:09:00 •Writing in completed square form: 00:07:00 •Solving by completed square method: 00:08:00 •Sketching of Quadratic Graphs: 00:12:00 •Quadratic graphs using Transformations: 00:06:00 •Quadratic inequalities: 00:11:00 •Deriving Quadratic formula: 00:05:00 •Solving problems using Quadratic Formula: 00:06:00 •Nature of Roots Part - 1: 00:05:00 •Nature of roots Part - 2: 00:12:00 •Downloadable Resources: 00:00:00 •Distance formula: 00:18:00 •Mid point formula: 00:05:00 •Gradient of a line: 00:11:00 •Graphing using gradient and y intercept: 00:03:00 •Some standard lines: 00:05:00 •Slope intercept form y = m x +c: 00:06:00 •Point slope form and two point form: 00:11:00 •Intersection of line and parabola: 00:10:00 •Past Papers Problems Part 1: 00:09:00 •Past Papers Problems Part 2: 00:11:00 •Past Papers Problems Part 3: 00:09:00 •Past Papers Problems Part 4: 00:12:00 •Past Papers Problems Part 5: 00:12:00 •Downloadable Resources: 00:00:00 •Sequence and series ( video): 00:08:00 •Arithmetic Sequence: 00:10:00 •General term of an A.P.: 00:07:00 •Finding given term is which term: 00:05:00 •Writing sequence when two terms are known: 00:08:00 •Condition for three terms to be in A.P.: 00:05:00 •Sum to n terms of A.P.: 00:06:00 •Practice Problems 1 (A.P.): 00:09:00 •Practice problems 2 (A.P.): 00:07:00 •Practice problems 3 (A.P.): 00:07:00 •Practice problems 4 (A.P.): 00:11:00 •Geometric Progressions: 00:12:00 •Sum to n terms in G.P.: 00:14:00 •Sum to infinite Terms in G.P.: 00:13:00 •Practice Problems 1 (GP): 00:15:00 •Practice Problems 2 (GP): 00:12:00 •Practice Problems 3 (GP): 00:07:00 •Practice Problems based on AP and GP both: 00:15:00 •Past papers problems 1: 00:17:00 •Past papers problems 2: 00:10:00 •Past papers problems 3: 00:11:00 •Downloadable Resources: 00:00:00 •Geometric Progressions - Resources: 00:00:00 •What is Factorial?: 00:07:00 •n-choose -r problems: 00:07:00 •Properties of n - choose -r: 00:05:00 •Binomial Theorem for positive index: 00:20:00 •Expanding using Binomial Theorem: 00:11:00 •Finding the indicated term in the Binomial expansion: 00:11:00 •Finding the indicated term from end: 00:09:00 •Finding the coefficient for given exponent (index) of the variable: 00:09:00 •Finding the term independent of variable: 00:05:00 •Expanding in increasing and decreasing powers of x: 00:09:00 •Practice problems 1: 00:12:00 •Practice Problems 2: 00:09:00 •Practice problems 3: 00:10:00 •Past papers problems 1: 00:15:00 •Past Paper problems 2: 00:13:00 •Past Paper problems 3: 00:09:00 •Downloadable Resources: 00:00:00 •What is Function?: 00:08:00 •Vertical Line Test: 00:04:00 •Value of a Function Graphically: 00:08:00 •Domain Range of a function Algebraically: 00:14:00 •Domain Range of a function Graphically: 00:07:00 •Even & Odd Functions: 00:07:00 •One to one Function: 00:05:00 •Composite Functions: 00:09:00 •How to draw Rational Functions- 1: 00:05:00 •How to draw Rational Functions- 2: 00:10:00 •Inverse of a function Algebraically: 00:05:00 •Inverse of a function Graphically: 00:09:00 •Practice Problems 1: 00:16:00 •Practice Problems 2: 00:11:00 •Downloadable Resources: 00:00:00 •What is Derivative?: 00:08:00 •Derivation of formula for Derivative: 00:06:00 •Differentiation by definition or First Principle: 00:07:00 •Power Rule: 00:22:00 •Practice Problems on Power Rule 1: 00:07:00 •Practice Problems on Power Rule 2: 00:07:00 •Practice Problems on Power Rule 3: 00:05:00 •Practice Problems on Power Rule 4: 00:13:00 •Practice Problems on Power Rule 5: 00:08:00 •Downloadable Resources: 00:00:00 •Tangents and Normals- Basics: 00:13:00 •Practice- Tangents and Normals Part 1: 00:16:00 •Practice- Tangents and Normals Part 2: 00:13:00 •Practice- Tangents and Normals Part 3: 00:11:00 •Practice- Tangents and Normals Part 4: 00:14:00 •Downloadable Resources: 00:00:00 •Stationary Points - Basics: 00:13:00 •Practice- Increasing Decreasing & Maxima Minima part 1: 00:11:00 •Practice- Increasing Decreasing & Maxima Minima part 2: 00:12:00 •Practice- Increasing Decreasing & Maxima Minima part 3: 00:10:00 •Downloadable Resources: 00:00:00 •Concavity-Basics: 00:02:00 •Concavity & Second Derivative: 00:08:00 •Second Derivative Test: 00:09:00 •Practice Problems on second derivative: 00:04:00 •Practice Problem of Maxima Minima using second derivative test Part 1: 00:17:00 •Practice Problem of Maxima Minima using second derivative test Part 2: 00:10:00 •Practice Problem of Maxima Minima using second derivative test Part 3: 00:07:00 •Practice Problem of Maxima Minima using second derivative test Part 4: 00:07:00 •Applications of Maxima and Minima Part 1: 00:09:00 •Applications of Maxima and Minima Part 2: 00:07:00 •Applications of Maxima and Minima Part 3: 00:10:00 •Applications of Maxima and Minima Part 4: 00:09:00 •Applications of Maxima and Minima Part 5: 00:10:00 •Applications of Maxima and Minima Part 6: 00:08:00 •Past Paper Problems on applications of maxima and minima Part 1: 00:09:00 •Past Paper Problems on applications of maxima and minima Part 2: 00:09:00 •Past Paper Problems on applications of maxima and minima Part 3: 00:08:00 •Past Paper Problems on applications of maxima and minima Part 4: 00:07:00 •Chain Rule: 00:12:00 •Rate of change part 1: 00:05:00 •Rate of change part 2: 00:10:00 •Rate of change part 3: 00:07:00 •Past Paper Problems using chain rule -1: 00:06:00 •Past Paper Problems using chain rule -2: 00:07:00 •Past Paper Problems using chain rule - 3: 00:07:00 •Past Paper Problems using chain rule - 4: 00:04:00 •Downloadable Resources: 00:00:00 •What is Integration?: 00:12:00 •Practice Questions 1: 00:06:00 •Practice Questions 2: 00:09:00 •Practice Questions 3: 00:09:00 •Fundamental Theorem of Calculus: 00:09:00 •What is Definite Integration?: 00:10:00 •Finding Definite Integration: 00:09:00 •Practice Questions on Definite Integration 1: 00:10:00 •Practice Questions on Definite Integration 2: 00:10:00 •Practice Questions on Definite Integration 3: 00:15:00 •Area below x-axis: 00:12:00 •Practice Problems on Area below x-axis 1: 00:11:00 •Practice Problems on Area below x-axis 2: 00:13:00 •Practice Problems on Area below x-axis 3: 00:09:00 •Practice Problems on Area below x-axis 4: 00:07:00 •Area between two curves (Basics): 00:15:00 •Practice Problems on Area between two curves 1: 00:06:00 •Practice Problems on Area between two curves 2: 00:13:00 •Practice Problems on Area between two curves 3: 00:12:00 •Practice Problems on Area between two curves 4: 00:10:00 •Practice Problems on Area between two curves 5: 00:13:00 •The Reverse Chain Rule- Indefinite Integration: 00:06:00 •The Reverse Chain Rule- Definite Integration: 00:05:00 •Practice Problems on The Reverse Chain Rule: 00:09:00 •Improper Integrals: 00:06:00 •Volumes by Integration: 00:08:00 •Practice Problems on Volumes by Integration-1: 00:04:00 •Practice Problems on Volumes by Integration-2: 00:04:00

Dive into the enthralling world of numbers and equations with 'High School Math (Pure Mathematics 1),' a course designed to unravel the mysteries of mathematics. Your journey begins with an Introduction that lays the foundation, not just in terms of concepts but igniting a passion for the beauty of math. As you progress, Functions become more than just equations; they turn into a language that describes the universe. Imagine the elegance of Quadratic Equations unfolding before your eyes, revealing patterns and solutions that were once hidden. Embark on an adventure through Co-ordinate Geometry, where every point and line tells a story of space and dimensions. Sequence and Series will no longer be just about numbers; they will be about the rhythm and flow of mathematical logic. The course takes a deeper dive with the Binomial Theorem, Differentiation, Tangents and Normals, each module building on the last, turning complexity into simplicity. Stationary Points & Curve Sketching, and the Second Derivative Test open new vistas in understanding the nature of graphs. As you master Simultaneous Linear Equations, you're not just solving problems; you're unlocking a new perspective on mathematical relationships. The Essential Revision at the end is your bridge to excellence, consolidating your knowledge and skills. Learning Outcomes Develop a foundational understanding of key mathematical concepts and functions. Master the intricacies of quadratic equations and co-ordinate geometry. Explore and apply the principles of sequences, series, and the binomial theorem. Gain proficiency in differentiation and its practical applications in tangents and normals. Understand and implement techniques in curve sketching, stationary points, and optimisation. Why choose this High School Math (Pure Mathematics 1) course? Unlimited access to the course for a lifetime. Opportunity to earn a certificate accredited by the CPD Quality Standards and CIQ after completing this course. Structured lesson planning in line with industry standards. Immerse yourself in innovative and captivating course materials and activities. Assessments designed to evaluate advanced cognitive abilities and skill proficiency. Flexibility to complete the Course at your own pace, on your own schedule. Receive full tutor support throughout the week, from Monday to Friday, to enhance your learning experience. Unlock career resources for CV improvement, interview readiness, and job success. Who is this High School Math (Pure Mathematics 1) course for? High school students seeking to excel in mathematics. Individuals preparing for college-level math courses. Math enthusiasts looking to deepen their understanding of pure mathematics. Students requiring a comprehensive revision of key mathematical concepts. Anyone aspiring to pursue a career involving advanced mathematics. Career path Mathematician: £30,000 - £60,000 Data Analyst: £25,000 - £50,000 Actuarial Analyst: £28,000 - £55,000 Research Scientist (Mathematics): £32,000 - £60,000 Engineering Consultant: £27,000 - £55,000 Academic Tutor (Mathematics): £24,000 - £40,000 Prerequisites This High School Math (Pure Mathematics 1) does not require you to have any prior qualifications or experience. You can just enrol and start learning.This High School Math (Pure Mathematics 1) was made by professionals and it is compatible with all PC's, Mac's, tablets and smartphones. You will be able to access the course from anywhere at any time as long as you have a good enough internet connection. Certification After studying the course materials, there will be a written assignment test which you can take at the end of the course. After successfully passing the test you will be able to claim the pdf certificate for £4.99 Original Hard Copy certificates need to be ordered at an additional cost of £8. Course Curriculum Introduction Introduction 00:03:00 Functions What is Function? 00:07:00 Vertical Line Test 00:04:00 Value of a Function Graphically 00:08:00 Domain Range of a function Algebraically 00:13:00 Domain Range of a function Graphically 00:06:00 Even & Odd Functions 00:07:00 One to one Function 00:05:00 Composite Functions 00:09:00 How to draw Rational Functions- 1 00:04:00 How to draw Rational Functions- 2 00:10:00 Inverse of a function Algebraically 00:05:00 Inverse of a function Graphically 00:09:00 Practice Problems 00:15:00 Practice Problems 00:11:00 Resources Downloads 00:40:00 Quadratic Equations Introduction to Quadratic Equations 00:04:00 Solving Quadratic Equations by Factorization method 00:10:00 Writing in completed square form 00:08:00 Solving by completed square method 00:08:00 Sketching of Quadratic Graphs 00:11:00 Quadratic graphs using Transformations 00:06:00 Quadratic inequalities 00:11:00 Deriving Quadratic formula 00:05:00 Solving problems using Quadratic Formula 00:06:00 Equations reducible to Quadratic 00:07:00 Nature of Roots of Quadratic Equations 00:04:00 Nature of roots continues 00:12:00 Quadratic Equations (Resources) 00:50:00 Co-ordinate Geometry Distance formula 00:15:00 Mid point formula 00:05:00 Gradient of a line 00:10:00 Graphing using gradient and y intercept 00:02:00 Some standard lines 00:04:00 Slope intercept form y = m x +c 00:05:00 Point slope form and two point form 00:10:00 Intersection of line and parabola 00:09:00 Practice Problems from past papers (part 3) 00:12:00 Sequence and series Sequence and series ( video) 00:08:00 Arithmetic Sequence 00:10:00 General term of an A.P. 00:07:00 Finding given term is which term? 00:05:00 Writing sequence when two terms are known 00:08:00 Condition for three terms to be in A.P. 00:05:00 Sum to n terms of A.P. 00:06:00 Practice Problems 1 (A.P.) 00:08:00 Practice problems 3 (A.P.) 00:07:00 Practice problems 4 (A.P.) 00:10:00 Geometric Progressions 00:11:00 Sum to n terms in G.P. 00:14:00 Sum to infinite Terms in G.P. 00:13:00 Practice Problems 1 (GP) 00:13:00 Practice Problems 2 (GP) 00:06:00 Practice Problems based on AP and GP both 00:15:00 Sequence and series Text 1 00:40:00 Sequence and series Text 2 00:55:00 Binomial Theorem What is Factorial? 00:06:00 n-choose -r problems 00:06:00 Properties of n - choose -r 00:05:00 Expanding using Binomial Theorem 00:11:00 Finding the indicated term in the Binomial expansion 00:10:00 Finding the indicated term from end 00:09:00 Finding the coefficient for given exponent (index) of the variable 00:08:00 Finding the term independent of variable 00:05:00 Expanding in increasing and decreasing powers of x 00:09:00 Practice problems 1 00:12:00 Practice Problems 2 00:09:00 Practice problems 3 00:10:00 Past papers problems 1 00:15:00 Past Paper problems 2 00:13:00 Past Paper problems 3 00:09:00 Resources in this section 00:50:00 Differentiation What is Derivative? 00:07:00 Derivation of formula for Derivative 00:06:00 Differentiation by definition or First Principle 00:06:00 Power Rule 00:20:00 Practice Problems on Power Rule 1 00:07:00 Practice Problems on Power Rule 2 00:07:00 Practice Problems on Power Rule 3 00:05:00 Practice Problems on Power Rule 4 00:11:00 Practice Problems on Power Rule 5 00:07:00 Tangents and Normals Tangents and Normals- Basics 00:12:00 Practice- Tangents and Normals Part 1 00:16:00 Practice- Tangents and Normals Part 2 00:13:00 Practice- Tangents and Normals Part 3 00:11:00 Practice- Tangents and Normals Part 4 00:14:00 Stationary Points & Curve Sketching Stationary Points - Basics 00:13:00 Practice- Increasing Decreasing & Maxima Minima part 1 00:11:00 Practice- Increasing Decreasing & Maxima Minima part 2 00:12:00 Practice- Increasing Decreasing & Maxima Minima part 3 00:10:00 Second Derivative Test (Maximum & Minimum Points) Concavity-Basics 00:02:00 Concavity & Second Derivative 00:08:00 Second Derivative Test 00:09:00 Practice Problems on second derivative 00:04:00 Practice Problem of Maxima Minima using second derivative test Part 1 00:17:00 Practice Problem of Maxima Minima using second derivative test Part 2 00:10:00 Practice Problem of Maxima Minima using second derivative test Part 3 00:07:00 Practice Problem of Maxima Minima using second derivative test Part 4 00:07:00 Applications of Maxima and Minima Part 1 00:09:00 Applications of Maxima and Minima Part 2 00:07:00 Applications of Maxima and Minima Part 3 00:10:00 Applications of Maxima and Minima Part 4 00:09:00 Applications of Maxima and Minima Part 5 00:10:00 Applications of Maxima and Minima Part 6 00:08:00 Past Paper Problems on applications of maxima and minima Part 1 00:09:00 Past Paper Problems on applications of maxima and minima Part 2 00:09:00 Past Paper Problems on applications of maxima and minima Part 3 00:08:00 Past Paper Problems on applications of maxima and minima Part 4 00:07:00 Chain Rule 00:12:00 Rate of change part 1 00:05:00 Rate of change part 2 00:10:00 Rate of change part 3 00:07:00 Past Paper Problems using chain rule -1 00:06:00 Past Paper Problems using chain rule - 2 00:07:00 Past Paper Problems using chain rule 3 00:07:00 Past Paper Problems using chain rule -4 00:04:00 Simultaneous Linear equations Graphical Method of solving pair of linear equations 00:10:00 Video lecture on Graphical method 00:05:00 Method of elimination by substitution 00:10:00 Video lecture on substitution method 00:06:00 Method of elimination by equating the coefficients 00:10:00 Video lecture on equating coefficients method 00:09:00 Practice Problems on Linear equation 00:20:00 Essential Revision How to take up this course? 00:10:00 Background of Algebra 00:10:00 Language of Alg ebra 00:10:00 Finding Values of algebraic expressions 00:14:00 Fractional Indices 00:10:00 Higher Indices 00:07:00 Rules of Brackets 00:04:00 Simplification by removing brackets (BODMAS) 00:11:00 Simplifications of Algebraic Fractions 00:07:00 Solving complex Linear Equations in one variable 00:10:00 Factorization by taking out common factor 00:10:00 Factorization by grouping the terms 00:09:00 Factorize using identity a ² - b ² 00:07:00 Factorization by middle term split 00:12:00

Functional Skills : Maths and English Level 2
By Course Cloud
Functional Skills: Maths and English Level 2 Course Overview Need a solid boost in both maths and English to get ahead in work or study? This Functional Skills: Maths and English Level 2 course has got you covered. From fractions and BODMAS to grammar and punctuation, we’ve packed everything into bite-sized lessons that are easy to follow and fun to complete. Whether you’re prepping for further education, looking to improve employability, or just brushing up on the basics, this course helps you feel confident with numbers and words — all at once. Plus, it's fully online, meaning you can learn at your own pace, from anywhere. Zero stress, just progress! Extra Included: Free Accredited Certificate Included Lifetime Access Free Student ID Card Learning Outcome Confidently handle maths concepts like decimals, percentages, ratios, and algebra Solve real-life word problems involving fractions, profit & loss, and scientific notation Read, write, and speak more clearly and correctly in English Spot and avoid common mistakes in writing Improve your academic, workplace, or everyday communication skills Who is this Functional Skills: Maths and English Level 2 Course for? This course is ideal for anyone looking to improve their everyday maths and English skills, whether you're aiming to meet entry requirements for further study, boost your CV, or gain confidence in practical tasks like budgeting, writing emails, or solving problems. It’s perfect for school leavers, job seekers, apprentices, and adults returning to education - basically, anyone who wants to sharpen their skills in a friendly, flexible way. Entry Requirements There are no formal requirements — just basic understanding of English and motivation to learn. Accredited Certification After successfully completing the course, you can get a UK and internationally accepted certificate to share your achievement with potential employers or include it in your CV. For the PDF certificate, you’ll need to pay £9.99. You can get the hard copy for 15.99, which will reach your doorstep by post.

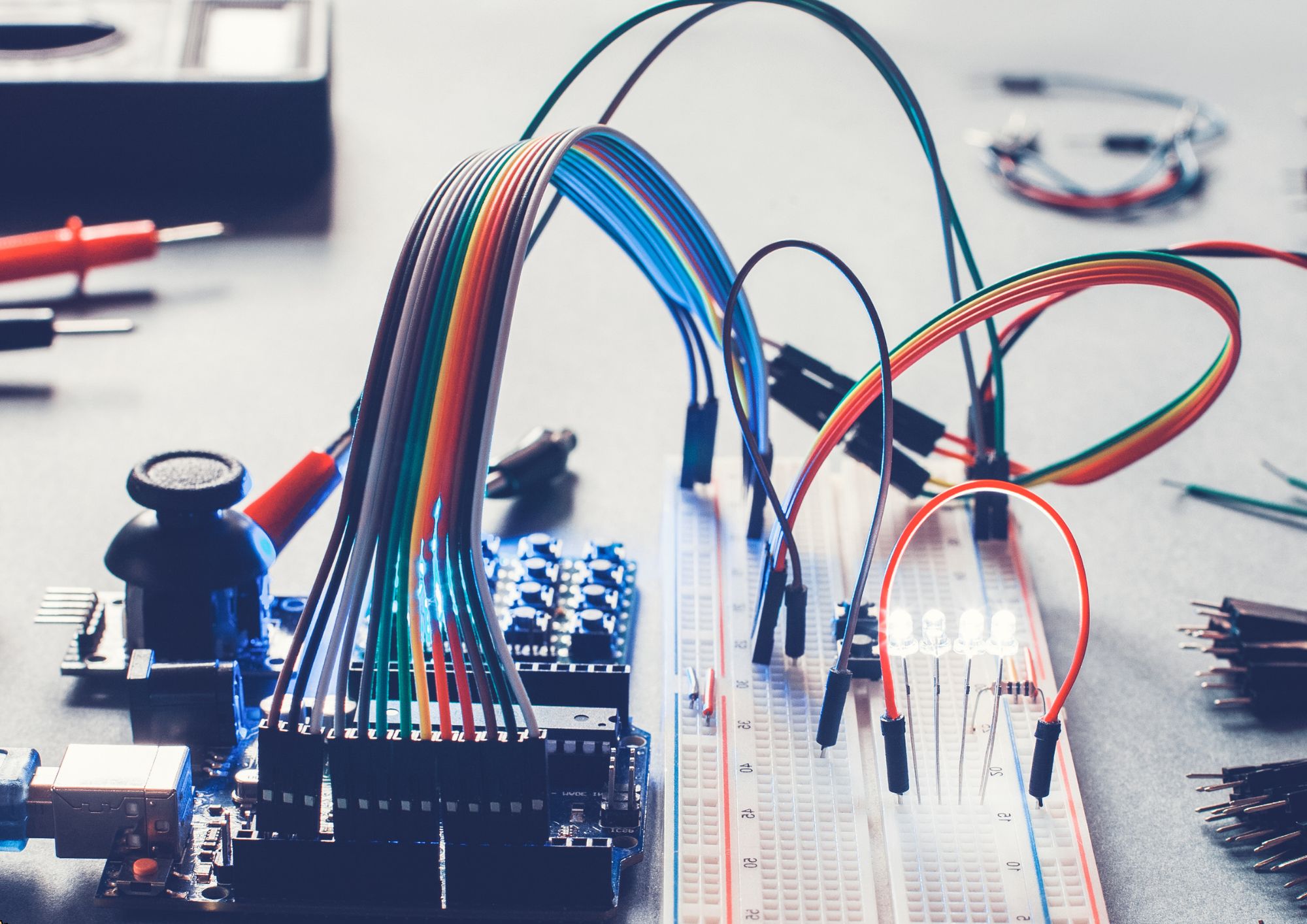
Diploma in Electrical Engineering
By Compete High
Boost your career with our Diploma in Electrical Engineering! ⚡ This self-paced online course covers essential topics like circuits, electrical machines, and power systems, helping you gain industry-relevant skills and a certificate of completion. Enrol today and take the next step in your Electrical Engineering journey! 🔌📚